Tubes Versus Transistors - Is There an Audible Difference?

by Russell O. Hamm

(Part 2 of 3 parts)

Go To Part 1
Go To Part 3
Back to "The Big Idea"

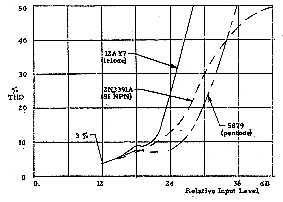
Single-stage amplifier comparison of total harmonic distortion (THD) graph

Fig. 2. Single-stage amplifier comparison of total harmonic distortion (THD).

The curves shown in Fig. 2 are representative of the general distortion characteristics of single-stage class A audio amplifiers. The devices are all operating open loop (no feedback) with a bias point which allows for maximum undis- torted output swing. The curves are referenced to a common point of 3% (THD), regardless of actual input or output levels. Since the objective of these comparisons is to detect variations in the slopes of the distortion character- istics, the x axis is a scale of relative level independent of circuit impedance considerations. These particular curves were chosen from the many plotted as representative of different families: silicon transistors, triodes, and pentode. A quick look shows that the often versed opinion that tubes overload more gently than transistors is obviously a myth.

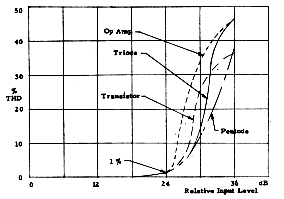
Multistage amplifier comparison of total harmonic distortion (THD) Graph

Fig. 3. Multistage amplifier comparison of total harmonic distortion (THD).

Fig. 3 shows the distortion characteristics for four differ- ent commercially available preamplifiers, using two or more stages of amplification. All the circuits use feedback, a couple are push-pull. Each amplifier is operating into 600 ohms at a gain of 40 dB. As in the previous curves, there is a common reference point of 1% THD. While these curves show a marked difference from the single-stage amplifiers, a review of the many different amplifiers tested shows that the slopes of all THD curves run about the same. The lack of a wide variation between the curves indicates that THD plots are not very relevant to what the ear hears in the listening tests.

Another series of tests were made on the same group of preamplifiers using a spectrum analyzer to measure the amplitude of individual harmonics. Each amplifier was driven 12 dB into overload, starting from a reference point of 1% third harmonic distortion. Every harmonic to the seventh was plotted. Since it is not possible to measure the relative phase of the harmonics on the spectrum analyzer, the over- load waveforms were recorded for Fourier analysis on the digital computer. The resulting plots divided amplifiers into three distinct categories.

1) Tube Characteristics

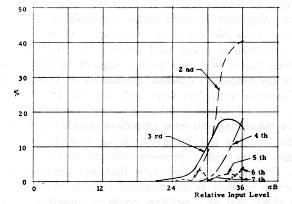
Distortion components for two-stage triode amplifier graph

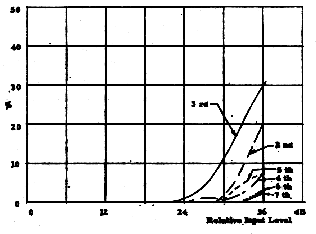
Fig. 4. Distortion components for two-stage triode amplifier.

Fig. 4 shows the distortion components for a typical two- stage 12AY7 amplifier. This particular design is quite representative of several single-ended, multistage triode tube amplifiers tested. The outstanding characteristic is the dominance of the second harmonic followed closely by the third. The fourth harmonic rises 3-4 dB later, running parallel to the third. The fifth, sixth, and seventh remain below 5% out to the 12-dB overload point. These curves seem to be a general characteristic of all the triode amplifiers tested, whether octal, miniature, nuvistor, single-ended, or push-pull. Fig. 5 is the waveform at 12 dB of overload. The clipping is unsymmetrical with a shifted duty cycle. Again this is a characteristic of all the triode amplifiers tested.

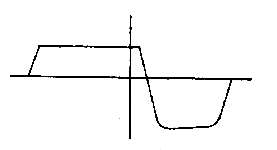
Waveform of triode amplifier of Fig. 4 at 12-dB overload. 1000-Hz tone

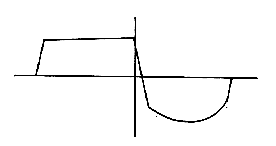
Fig. 5. Waveform of triode amplifier of Fig. 4 at 12-dB overload. 1000-Hz tone

Distortion components for two-stage pentode amplifier (graph)

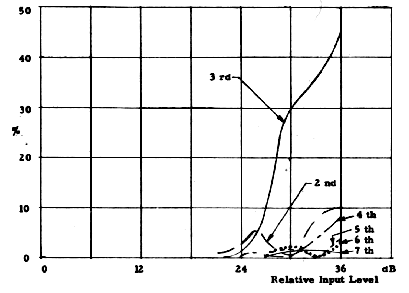
Fig. 6. Distortion components for two-stage pentode amplifier.

Fig. 6 shows the distortion components for a two-stage single-ended pentode amplifier. Here the third harmonic is dominant and the second rises about 3 dB later with the same slope. Both the fourth and fifth are prominent while the sixth and seventh remain under 5%. The waveform at 12-dB overload (Fig. 7), is similar to the triode, but its duty cycle is not shifted as much. It is not reasonable to assume that virtually all tube amplifiers can be represented by these two examples. However, the major characteristic of the tube amplifier is the presence of strong second and third harmonics, sometimes in concert with the fourth and fifth, but always much greater in amplitude. Harmonics higher than the fifth are not significant until the overload is beyond 12 dB. These characteristics seem to hold true for wide variations in circuit design parameters. The extreme difference in the tube amplifiers is the interchanging of the position of the second and third harmonics. This effect is not just a characteristic of the pentode, it is common to triodes too.

Waveform of pentode amplifier of Fig. 6 at 12 dB overload, 1000-Hz tone

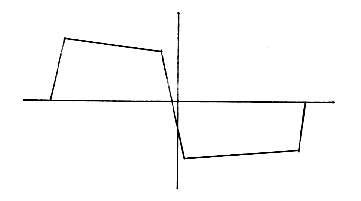
Fig. 7. Waveform of pentode amplifier of Fig. 6 at 12 dB overload, 1000-Hz tone.

Distortion components for multistage capacitor-coupled transistor amplifier (graph)

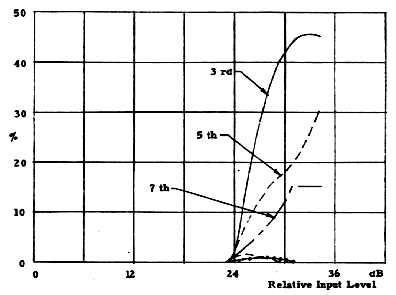
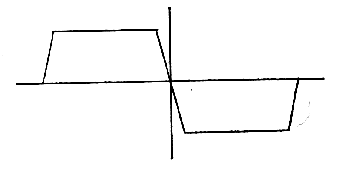
Fig. 8. Distortion components for multistage capacitor-coupled transistor amplifier.

2) Transistor Characteristics

Figs. 8 and 10 show the characteristics of two transistor amplifiers. Like the previous figures the curves are representative of all the transistor amplifiers tested. The distinguishing feature is the strong third harmonic component. All other harmonics are present, but at a much lower amplitude than the third. When the overload reaches a break point, all the higher harmonics begin to rise simultaneously. This point is generally with 3-6 dB of the 1% third harmonic point. The waveforms of these amplifiers (Figs. 9 and 11) are distinctly square wave in form with symmetrical clipping and an almost perfect duty cycle. Both amplifiers shown have single-ended inputs and push-pull outputs. However, the circuit designs are radically different.

Waveform for transistor amplifier of Fig. 8 at 12-dB overload, 1000-Hz tone

Fig. 9. Waveform for transistor amplifier of Fig. 8 at 12-dB overload, 1000-Hz tone.

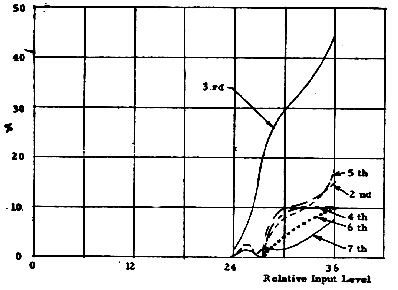
Distortion components for multistage transformer-coupled transistor amplifier (graph)

Fig. 10. Distortion components for multistage transformer-coupled transistor amplifier.

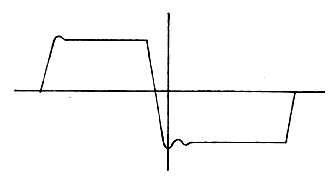
Waveform for transistor amplifier of Fig. 10 at 12-dB overload, 1000-Hz tone

Fig. 11. Waveform for transistor amplifier of Fig. 10 at 12-dB overload, 1000-Hz tone.

3) Operational-Amplifier Characteristics

Fig. 12 is a hybrid operational amplifier. The third harmonic rises steeply as the dominant distortion component in a characteristic similar to the transistor. Also rising very strongly from the same point are the fifth and seventh harmonics. All even harmonics are suppressed completely. The waveform of Fig. 13 is a perfect square wave. As a classification group, operational amplifiers have the most uniform characteristics with almost no deviation from the curves shown in this example.

Distortion components for monolithic operational amplifier with hybrid output stage (graph)

Fig. 12. Distortion components for monolithic operational amplifier with hybrid output stage.

Waveform for operational amplifier of Fig. 12 at 12-dB overload, 1000-Hz tone

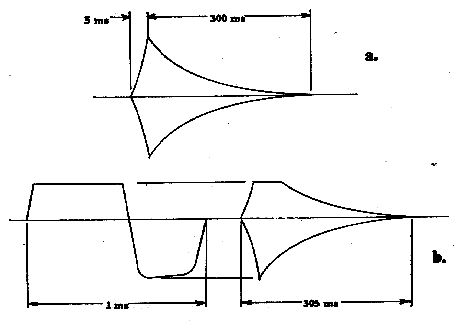
Fig. 13. Waveform for operational amplifier of Fig. 12 at 12-dB overload, 1000-Hz tone.

In view of the transient nature of audio signals, steady- state single-frequency distortion analysis could yield questionable results. Indeed, the arguments for and against sine-wave and pulse test signals for audio system testing have been the subject for a number of engineering papers [4], [7]. For our purposes, however, a few minutes toying with an electronic synthesizer quickly proved that musical instruments do not produce fast pulses. For example, a good simulation of the large floor tom used in the amplifier listening tests is a 100-Hz tone modulated with an envelope rise time of 5 ms and a decay time of 300 ms. Also an extensive study of trumpet tones [6] measured the rise time of the fastest staccato notes at 12 ms. Certainly, rise times of these orders can not be considered pulses for audio amplifiers with passbands extending to 20 kHz or better. Just to further prove the correctness of the preceding steady-state results, the synthesized floor tom signal was used to test the same amplifiers at the same level as the microphone signal.

Envelope of Moog-generated floor tom test signal & envelope clipping

Fig. 14. a. Envelope of Moog-generated floor tom test signal. b. Envelope clipping of transient signals by amplifier is identical to single-frequency clipping levels.

Careful observation of the amplified signal showed that envelope clipping was identical to the steady-state clipping level (Fig. 14). There were no glitches or other fast transient phenomena in the output signal.

SIGNIFICANCE OF MUSICAL HARMONICS

Having divided amplifiers into three groups of distortion characteristics, the next step is to determine how the harmonics relate to hearing. There is a close parallel here between electronic distortion and musical tone coloration that is the real key to why tubes and transistors sound different. Perhaps the most knowledgeable authorities in this area are the craftsman who build organs and musical instruments [8], [9]. Through many years of careful experimentation these artisans have determined how various harmonics relate to the coloration of an instrument's tonal quality.

The primary color characteristic of an instrument is determined by the strength of the first few harmonics. Each of the lower harmonics produces its own characteristic effect when it is dominant or it can modify the effect of another dominant harmonic if it is prominent. In the simplest classification, the lower harmonics are divided into two tonal groups. The odd harmonics (third and fifth) produce a "stopped" or "covered" sound. The even harmonics (second, fourth, and sixth) produce "choral" or "singing" sounds.

The second and third harmonics are the most important from the viewpoint of the electronic distortion graphs in the previous section. Musically the second is an octave above the fundamental and is almost inaudible; yet it adds body to the sound, making it fuller. The third is termed quint or musical twelfth. It produces a sound many musicians refer to as "blanketed." Instead of making the tone fuller, a strong third actually gives the sound a metallic quality that gets annoying in character as its amplitude increases. A strong second with a strong third tends to open the "covered" effect. Adding the fourth and fifth to this changes the sound to an "open horn" like character.

The higher harmonics, above the seventh, give the tone "edge" or "bite." Provided the edge is balanced to the basic musical tone, it tends to reinforce the fundamental, giving the sound a sharp attack quality. Many of the edge harmonics are musically unrelated pitches such as the seventh, ninth, and eleventh. Therefore, too much edge can produce a raspy dissonant quality. Since the ear seems very sensitive to the edge harmonics, controlling their amplitude is of paramount importance. The previously mentioned study of the trumpet tone [6] shows that the edge effect is directly related to the loudness of the tone. Playing the same trumpet note loud or soft makes little difference in the amplitude of the fundamental and the lower harmonics. However, harmonics above the sixth increase and decrease in amplitude in almost direct proportion to the loudness. This edge balance is a critically important loudness signal for the human ear.

(Click here to continue reading this article)
Back to "The Big Idea"